Social Phenomena Overlaying Thermodynamics (7)

Let's begin the process of creating expressions to give a form to the problem statement.

Let us denote by Expression 1 the expression “to find the coordinates of a shared point.”

Looking at the graph, Expression 1, “Find the coordinates of the shared point,” can be transformed into Expression 2, “Find the X and Y of the intersection".
The expression “Find the coordinates” is transformed into the Expression 2.

The values of X and Y at the intersection satisfy the two equations y=-x²+2x+3 and y=-2x-2.
Then, X and Y satisfying the two equations is equivalent “to finding X and Y satisfying the simultaneous equations.”, Expression 3.

Since the student has learned to solve the simultaneous equations in Expression 3,
he can calculate Y = 0 when X = -1 and Y = -12 when X = 5 that is Expression 4.

The coordinates (X,Y) of the shared point can be written as (-1, 0) (5, -12) that is Expression 5.

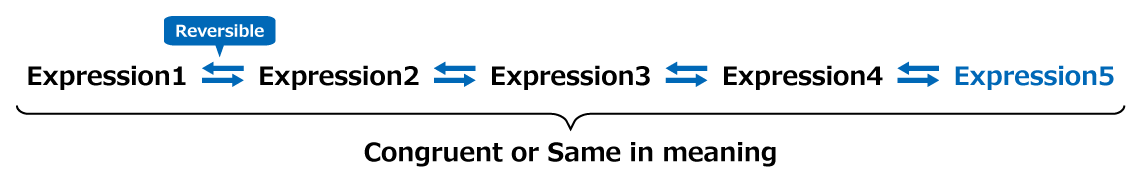
Expression 1 through Expression 5 can be transformed without changing their value (meaning).
That is, the expressions are reversible. The answer is a simple Expression 5.

The problem statement was positioned as a truth, which was then transformed into Expression 1 to Expression 5.
Expression 5 was the answer.
It is possible to go backwards from Expression 5 to Expression 1 (it is reversible).
That is, the values of Expression 1 to Expression 5 are the same (congruent).

In this case, the truth, which appears to be complex, is the problem statement and the simple expression is the answer.
There are many possible types of reversible expressions. Reversible expressions are congruent.
If an expression expresses truth, then truth has many expressions.

[ Author : Y. F. ]